Communications of the ACM
How Did the Romans Calculate?

Today we use a place value system and Indo-Arabic numerals (0 to 9) for calculations. The Romans utilized number symbols like I, V, X, L, C, D, M. They had no sign for zero and no negative numbers. Therefore there is no year 0.
Experts are still wondering how the Romans calculated with their number system. No document is known that describes its handling. Dealing with their numerals is considered difficult. However, it is unbelievable that the Roman engineers constructed great buildings and that the merchants did a lot of trading without being able to calculate.
Chinese, Japanese, and Russian Abacus
The most natural tool for counting and calculating are the fingers. The hands can be used to represent large numbers. For arithmetic, among other things, shells and pebbles were used. There were different types of counting boards and bead frames. The chronological development goes from the Babylonian abacus to Greek and Roman calculating boards. This was followed by the Chinese, Japanese and Russian abacus and finally the teaching abacus. Whether the Chinese took over the Roman bead frame or invented it independently is unclear. It is also uncertain whether the Japanese abacus derives from the Chinese.
The bead frames were used for centuries, but today they have largely disappeared. You could calculate on that very quickly. Addition and subtraction are comparatively simple, multiplication (repeated addition) and division (repeated subtraction) more difficult. With beads, addition and subtraction can be traced back to counting. In the Roman hand abacus one should actually speak of buttons and not of beads.
Roman hand abacus
The Romans had a portable "pocket calculator", the hand abacus (see Fig. 1–2). To the best of our knowledge, only three original copies have survived (Aosta, Paris, Rome). In addition, there are some drawings and several replicas (some from unknown manufacturers). Most of the devices were probably made of wood, therefore they did not survive. Moreover, there was apparently also a larger version, but there are no copies.

Fig. 1: Roman hand abacus (replica).
The device uses the decimal system (lower part) and the quinary system (upper part).
The value shown here is 5 555 555 as and 7 ounces.
(courtesy of Braunschweigisches Landesmuseum, Braunschweig, Picture: Anja Pröhle)
The Roman hand abacus has seven decimal columns. Below are the ones (I), tens (X), hundreds (C) etc. The upper beads each have five times the value. For the thousands, the character φ was originally used. The number M was introduced only in the Middle Ages. The slot marked with a circle ○ is used for the ounces. Since 1 as (pound) has 12 ounces, this slot contains five single beads at the bottom and a six beads at the top. Thus 5+6=11 ounces can be represented. The right-most groove might have been used for ½, ¼, and ⅓ ounces.

Fig. 2: Modern mass-produced replica of a Roman hand abacus.
(courtesy of Alain Schärlig. Location of the object: Adam-Ries-Museum, Annaberg-Buchholz)
This replica corresponds to two drawings from the 17th century made in Augsburg and Rome. They are probably true to life, but the originals are lost. The reconstruction differs from the only three preserved original copies in that the ounce fractions in the right-most slot are represented differently. In this calculator the right column is divided into three parts.
In the basic position the beads are in the lower section (hell) below, in the upper section (heaven) above. A bead at the center bar has the value 1, 10, 100 (hell) etc. or 5, 50, 500 (heaven) etc. Detailed step-by-step instructions can be found in my book "Meilensteine der Rechentechnik" (Milestones in analog and digital computing).
Numerical notation
The numbers are displayed on the Roman reckoner in a similar way to the Chinese and Japanese models (see Fig. 3).
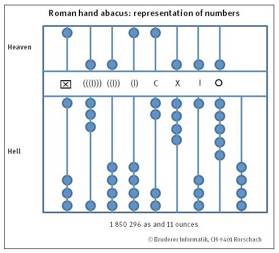
Fig. 3: Numerical notation.
The Romans did not have a symbol for the zero, but could represent the zero (= nothing) on the abacus.
The bead frame can also be used to represent the place value system.
It is generally assumed that the beads in the right-most column have the values ½ (top), ¼ (middle), and ⅓ (bottom) (see Fig. 4). If the right column is one-piece, the handling is a bit confusing, with three parts it is easier.

Fig. 4: Fractions on the Roman hand abacus.
In this drawing there are three separate slots.
These values can also be shown on a single groove.
The assumption that the two beads below each have the value of ⅓ has the consequence from today's point of view that
- the following fractions cannot be displayed: 1/12, 2/12 and 5/12,
- the following fractions above 12/12 can be shown: 13/12, 14/12 and 17/12 (see Fig. 5).
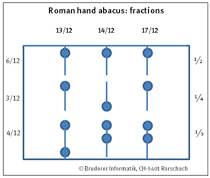
Fig. 5: Values that make little sense.
It is hardly true that the two lower beads each have the value ⅓.
If one assigns the value 1/12 to each of the two lowest beads, a seemingly meaningful solution results (see Fig. 6). Thus all fractions from 1/12 to 11/12 can be set without gaps.

Fig. 6: Representation of all fractions from 1/12 to 11/12.
The lowest beads don't have the value of 1/12
For the ounce fractions (last slot) the ounce slot (penultimate slot) could have been repeated. Because with it all fractions from 1/12 to 11/12 could be (much easier) represented. It is therefore unlikely that the lowest beads have the value of 1/12. On the Roman hand abacus, fractions can be represented, but they are not compatible with the Roman numbering system.
The use of the last column remains a mystery
Alain Schärlig and Jérôme Gavin, both historians of mathematics, think that the solution presented in Wikipedia is wrong. It is uncertain what the characters next to the last slot stand for. In the Roman currency, by the way, there were only half ounces. Due to the devaluation of money, a twelfth ounce would have had only a very small value anyway.
How did the Romans calculate with their numeral system?
I believe that the Romans calculated with the abacus and only used the Roman numerals to record the results. The experience with the Chinese, Japanese and Russian abacus as well as the school counting frame shows that one can calculate very fast with these instruments. Addition and subtraction are simple, but multiplication and division are more demanding.
References
Bruderer, Herbert: Meilensteine der Rechentechnik (Milestones in analog and digital computing), De Gruyter Oldenbourg, Berlin/Boston 2018, https://www.degruyter.com/view/product/480555 (volume 1), https://www.degruyter.com/view/product/503373 (volume 2).
Bruderer, Herbert: Zeitrechnung. Wie genau ist unser Kalender? Warum gibt es in der christlichen Zeitrechnung kein Jahr Null? (Time reckoning. How exact is our calendar? Why is there no zero year in the Christian calendar?) 2011, https://doi.org/10.3929/ethz-a-006450534
Gavin, Jérôme, personal communication of 18 February 2019
Igarashi, Yoshihide; Altman, Tom; Funada, Mariko; Kamiyama, Barbara: Computing. A historical and technical perspective, CRC press, Boca Raton 2014
Karcher, Hermann: Konnten die Römer wirklich nicht rechnen?, Mitteilungen der DMV, 2014, https://www.degruyter.com/downloadpdf/j/dmvm.2014.22.issue-3/dmvm-2014-0056/dmvm-2014-0056.pdf
Karcher, Hermann; Gansohr-Meinel, Heidrun: Der Abakus ̶ völlig unterschätzt, in: epoc, 2012, https://www.spektrum.de/pdf/77-77-epoc-01-2012-pdf/1142345?file
Schärlig, Alain: Compter avec des cailloux. Le calcul élémentaire sur l'abaque chez les anciens Grecs, Presses polytechniques et universitaires romandes, Lausanne 2001
Schärlig, Alain: Compter avec des jetons. Tables à calculer et tables de compte du Moyen Age à la Révolution, Presses polytechniques et universitaires romandes, Lausanne 2003
Schärlig, Alain, personal communication of 18 February 2019
https://en.wikipedia.org/wiki/Roman_abacus
https://ethw.org/Ancient_Computers
https://www.ee.ryerson.ca/~elf/abacus/history.html
Herbert Bruderer is a retired lecturer in didactics of computer science at ETH Zürich. More recently, he has been an historian of technology. bruderer@retired.ethz.ch, herbert.bruderr@bluewin.ch
No entries found